Solving Absolute Value Equations
Recommended for You
Not sure where to start? These are the lessons and games we recommend most to students, parents and teachers on Basic-mathematics.com. Each lesson explains the topic in plain language, walks you through worked examples step by step, and ends with practice so you can check your understanding. Pick one below, or use the menu to find lessons on fractions, algebra, geometry, statistics and more.
When solving absolute value equations, many people routinely solve these as the example below demonstrates without really understand deep concepts involving absolute value. It is the goal of this lesson to remedy this common pitfall.
The goal is to use the absolute value definition to solve absolute value equations
Absolute value definition:
If x is positive, | x | = x
If x is negative, | x | = -x
Example #1:
Solve for x when | x | = 4
After applying the definition to example #1, you will have two equations to solve.
In fact, when solving absolute value equations, you will usually get two solutions. That is important to keep in mind.
If x is positive, | x | = x, so the first equation to solve is x = 4. We are done because x is automatically isolated.
If x is negative, | x | = -x, so the second equation to solve is -x = 4.
You can write -x = 4 as -1x = 4 and divide both sides by -1 to isolate x.
(-1 / -1)x = 4 / -1
1x = -4
x = -4
Therefore, the solutions are 4 and -4
Example #2:
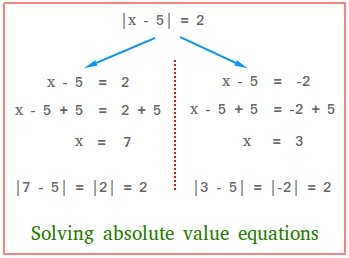
Solve for x when | x − 5 | = 2
Before we apply the definition, let's make a useful substitution.
Let y = x − 5, so | x − 5 | = 2 becomes | y | = 2. You must understand this step. No excuses!
Now, let's apply the definition to | y | = 2. Again, you will have two equations to solve
Once again, when solving absolute value equations, you will usually get two solutions.
If y is positive, | y | = y, so the first equation to solve is y = 2. No, you are not done! You have to substitute x − 5 for y.
After substitution, y = 2 becomes x − 5 = 2
x − 5 = 2
x + -5 = 2
x + -5 + 5 = 2 + 5
x = 7
If y is negative, | y | = -y, so the second equation to solve is -y = 2.
You have to substitute x − 5 for y
You get -(x − 5) = 2. Notice the inclusion of parenthesis this time.
-(x − 5) = 2
Multiply both sides by -1
-1 × -(x + -5) = -1 × 2
x + -5 = -2
x + -5 + 5 = -2 + 5
x + 0 = -2 + 5
x = 3
The solutions are 3 and 7
Example #3:
Solve for x when | 3x + 3 | = 15
Before, we apply the definition, let's again make a useful substitution
Let y = 3x + 3, so | 3x + 3 | = 15 becomes | y | = 15.
Now, let's apply the definition to | y | = 15. Again, you will have two equations to solve.
If y is positive, | y | = y, so the first equation to solve is y = 15. You have to substitute 3x + 3 for y.
After substitution, y = 15 becomes 3x + 3 = 15
3x + 3 = 15
3x + 3 − 3 = 15 − 3
3x = 12
(3 / 3)x = 12 / 3
x = 4
If y is negative, | y | = -y, so the second equation to solve is -y = 15.
You have to substitute 3x + 3 for y.
You get -( 3x + 3) = 15. Notice the inclusion of parenthesis this time.
-3x + -3 = 15
-3x + -3 + 3 = 15 + 3
-3x = 18
(-3 / -3)x = 18 / -3
x = -6
The solutions are -6 and 4
Solving absolute value equations should be straightforward if you follow my guidelines above.
Share this page