Area of a kite

To find the area of a kite, we will use the kite below with a line of symmetry d₁. Notice that when d₁ is a line of symmetry, the kite is made of 2 congruent triangles.

Area of kite  =  area of triangle ABC + area of triangle ADC

Be careful!

base = d₁







base = d₁

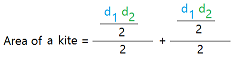
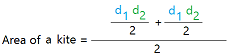
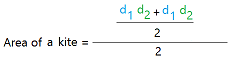
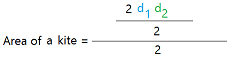
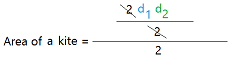
Here is the formula for the area of a kite using the diagonals.

Once you know the lengths of the diagonals, you can just multiply them and divide the result by 2.

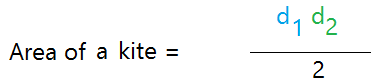
Example #1

Find the area of a kite with diagonals that are 6 inches and 18 inches long.

Area  = (6 × 18) / 2 = 108 / 2 = 54 square inches.

Example #2

When the diagonals of a kite meet, they make 4 segments with lengths 6 meters, 4 meters, 5 meters, and 4 meters. What is the area of the resulting kite.

The segments with lengths 4 meters and 4 meters must represent the segment that was bisected into 2 equal pieces or d₂

d₂ = 4 + 4 = 8 meters

The segments with lengths 6 meters and 5 meters must represent d₁ then

d₁ = 6 meters + 5 meters = 11

Area = (8 × 11) / 2 = 88 / 2 = 44 square meters

Another formula for the area of a kite using trigonometry.

This concept is based on finding the area of a triangle given sas (side-angle-side).

For example, looking at the figure above, two unequal sides could be CB and AB. The angle between them is angle B.

The area of triangle ABC is 1/2[CB × AB × sin(B)]

Since diagonal d₁ is a line of symmetry, triangle ADC and triangle ABC are congruent triangles.

Therefore, the area of triangle ADC is equal to the area of triangle ABC. The total area is found by adding the areas of the two triangles.

Area of the kite = area of triangle ABC  + area of triangle ADC

Area of the kite = 1/2[CB × AB × sin(B)]  + 1/2[CB × AB × sin(B)]

Area of the kite = [CB × AB × sin(B)] (1/2 + 1/2) 

Area of the kite = [CB × AB × sin(B)] (1) 

Area of the kite = [CB × AB × sin(B)]

Example #3

Suppose CB = 8 cm, AB = 15 cm, and angle B is 90 degrees. Find  the area of the kite.

Area of the kite = [8 × 15 × sin(90 degrees)]

Area of the kite = [120 × sin(90 degrees)]

Area of the kite = (120 × 1)

Area of the kite = 120 cm²

A rhombus is a kite made with two isosceles triangles.

Example #4

Find the area of the kite shown in the figure below which can also be called a rhombus.

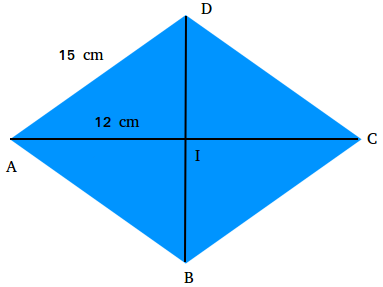
AD = 15 cm and AI  = 12 cm

Use the Pythagorean theorem to find the length of segment DI

15² = 12² + DI²

225 = 144 + DI²

225 - 144 = 144 - 144 + DI²

225 - 144 = 144 - 144 + DI²

81 = DI²

9 = DI

Since the diagonals of a rhombus bisect each other, AC = 24 and DB = 18.

According to the formula, we know that the area is half the product of the lengths of its diagonals.

Area  = 1/2(24 × 18)

Area  = 1/2(432)

Area  = 216 cm²