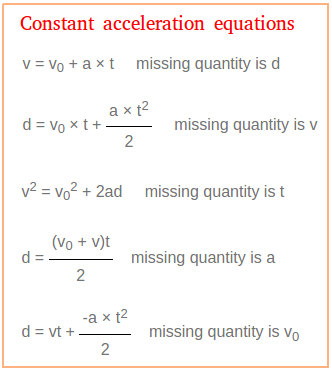
Constant acceleration equations
To derive the constant acceleration equations, we will need the following free fall equations.
v = v0 + g × t
d = v0 × t +
g × t2
2
Remember that g is the acceleration of gravity and it is a constant acceleration. It is not only gravity that can give a constant acceleration. A car can drive also with a constant acceleration. We can generalize the two equations above by replacing g with a.
a is this case is any constant acceleration.
v = v0 + a × t equation 1
d = v0 × t +
a × t2
2
equation 2Derivation of more constant acceleration equations
We can use the equations above to get 3 more constant acceleration equations. To derive the constant acceleration equations, concepts of factoring, simplifying exponents, and fractions will be used.
Solve for t in equation 1
v = v0 + a × tv - v0 = a × t
t =
v - v0
a
Rewrite equation 2
d =
2v0 × t + at2
2
2d = 2v0 × t + at2 (equation a)
Replace t in equation a
|
2d = 2v0
v - v0
a
|
+ a
(v- v0)2
a2
|
|
2d = 2v0
v - v0
a
|
+
(v- v0)2
a
|
|
2d = 2v0
v - v0
a
|
+ a
(v- v0)2
a2
|
|
2d = 2v0
v - v0
a
|
+
(v- v0)2
a
|
2d =
v - v0
a
(2v0 + v - v0)
2d =
v - v0
a
(v + v0)
2d =
v2 - v02
a
v2 - v02 = 2ad
v2 = v02 + 2ad equation 3
Solve for a in equation 1.
v = v0 + a × tv - v0 = a × t
a =
v - v0
t
Equation 2 can also be rewritten as
d = v0 × t + a ×
t2
2
equation 2Replace a in the latter equation 2
|
d = v0t +
v - v0
t
|
×
t2
2
|
Replace a in the latter equation 2
|
d = v0t +
v - v0
t
|
×
t2
2
|
d = v0t +
(v - v0)t
2
d =
2v0t + vt - v0t
2
d =
v0t + vt
2
d =
(v0 + v)t
2
equation 4
Finally, solve for v0 in equation 1 and replace v0 in equation 2.
v0 = v - at
d = (v - at) × t +
a × t2
2
d = vt - at2 +
a × t2
2
d = vt +
-2at2 + a × t2
2
d = vt +
-a × t2
2
equation 5
We then have five important constant acceleration equations
Any questions about how I derive the constant acceleration equations, send me an email.