Definition of Absolute Value

Recommended Lessons

Not sure where to start? These are the lessons and games we recommend most to students, parents and teachers on Basic-mathematics.com. Each lesson explains the topic in plain language, walks you through worked examples step by step, and ends with practice so you can check your understanding. Pick one below, or use the menu to find lessons on fractions, algebra, geometry, statistics and more.

Definition of Absolute Value

In this lesson, you will learn

  • what absolute value means: the distance of a number from zero
  • why an absolute value is never negative
  • the formal definition: |x| = x or |x| = −x
  • how to find the absolute value of a numerical expression

Absolute Value Is the Distance From Zero

Definition

The absolute value of a number is the distance the number is from zero.

Take a look at the following two graphs: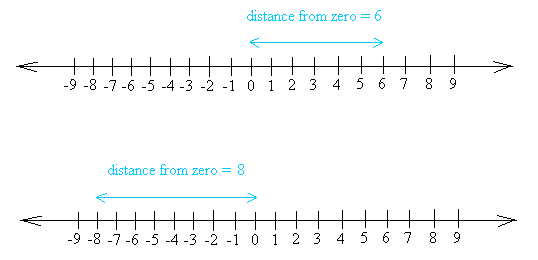

Two number lines from −9 to 9. On the first, an arrow from 0 to 6 shows a distance from zero of 6. On the second, an arrow from 0 to −8 shows a distance from zero of 8.

The first graph shows 6 located at a distance of 6 units from zero.

Then, the absolute value of 6 is 6 and we write |6| = 6

The second graph shows −8 located at a distance of 8 units from zero.

Then, the absolute value of −8 is 8 and we write |−8| = 8.

You can see from this that the absolute value of a number is always positive with the exception of taking the absolute value of 0 (|0| = 0)

Common mistake

Therefore, do not write |5| = −5 or |4| = −4 please!

|6| means distance from 0 to 6.

|−8| means distance from 0 to −8.

Which two numbers have an absolute value of 7?

Show answer

7 and −7. Both are 7 units from zero, one on each side.

The Formal Definition of Absolute Value

For |−8| = 8, you could also argue that to get 8, you have to take the negative of −8 since −(−8) = 8

So, |−8| = −(−8) = 8.

This observation helps us to come up with a formal definition of absolute value.

Definition

|x| = x if x is positive or zero, but −x if x is negative.

This definition is important to understand before solving absolute value equations or absolute value inequalities.

Remember

When x is negative, −x is positive. For example, if x = −3, then −x = −(−3) = 3.

Use the formal definition to find |−12|.

Show answer

12. −12 is negative, so |−12| = −(−12) = 12.

Absolute Value of Numerical Expressions

Calculate the absolute value of the following numerical expressions.

Rule

First work out the expression inside the bars, using the order of operations. Then take the absolute value of the result.

1) −8 + 2 × 5

|−8 + 2 × 5| = |−8 + 10|

|−8 + 2 × 5| = |2|

|−8 + 2 × 5| = 2

2) 42 − 4 × 2

|42 − 4 × 2| = |16 − 4 × 2|

|42 − 4 × 2| = |16 − 8|

|42 − 4 × 2| = |8|

|42 − 4 × 2| = 8

3) −5 + 5 × 2 − 15

|−5 + 5 × 2 − 15| = |−5 + 10 − 15|

|−5 + 5 × 2 − 15| = |5 − 15|

|−5 + 5 × 2 − 15| = |−10|

|−5 + 5 × 2 − 15| = 10

Worked example: Find |3 − 4 × 5|.
  1. Multiply first: 4 × 5 = 20, so the expression is |3 − 20|.
  2. Subtract: 3 − 20 = −17, so you have |−17|.
  3. −17 is 17 units from zero, so |−17| = 17.

Answer: 17

Common mistake

Making each number positive before you calculate. For |−8 + 2 × 5|, writing 8 + 10 = 18 is wrong. Work out −8 + 10 = 2 first, then |2| = 2.

Find |2 × 3 − 10|.

Show answer

4. 2 × 3 − 10 = 6 − 10 = −4, and |−4| = 4.

Summary

  • The absolute value of a number is its distance from zero.
  • An absolute value is never negative: it is positive, or 0 when the number is 0.
  • A number and its opposite have the same absolute value, for example |6| = |−6| = 6.
  • Formal definition: |x| = x if x ≥ 0, and |x| = −x if x < 0.
  • For an expression, work out the inside first, then take the absolute value.

Test Yourself: 10-Question Quiz

Now check what you learned. Every answer is explained, and each time you play you get new questions.

Question 1 of 10Score: 0

Keep learning