Direct variation

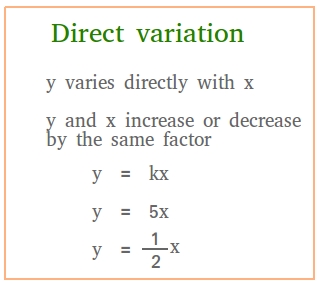
A direct variation, also called direct proportion is a relationship between two variables x and y that can be written as y = kx, k ≠ 0.

This situation occurs when the ratio of two variables is constant.

When y = kx, we say that y varies directly with x.

When z = kt, we say that z varies directly with t.

k is called the constant of variation.

Take a close look at the figure below and then read the real life example of direct variation

Direct variation
You are probably familiar with lighting. The distance that you are from lighting and the time it takes you to hear thunder could form a direct proportion.

Let's say you are 4 miles away from lighting and it takes you 20 seconds to hear thunder. And when you are 5 miles away, it takes you 25 seconds.

The ratio of the time it takes to distance you are from lighting is constant since 20/4 = 25/5 = 5.

The situation above represents a direct proportion and you can find the equation that relates the time it takes to hear the thunder and the distance you are from lighting.

Let x = distance from lighting and let y = time it takes to hear the thunder.

The time it takes to hear the thunder depends on the distance you are. Thus, the time it takes to hear thunder varies directly with the distance you are.

Then y = kx

Find k when x = distance from lighting = 4 and y = time it takes to hear thunder = 20.

20 = k × 4

20/4 = k

5 = k

Therefore, y = 5x is the direct variation equation.

Having that relationship is a good thing since you could now predict how long it will take to hear the thunder if you are 10 miles away. 

When x = 10, y = 5 × 10 = 50 seconds.

Another real life example of direct variation about recipe.


Example #2:

A recipe for 6 cupcakes needs 1 cup of flour. The number of cupcakes you can make varies directly with the amount of flour. How many cupcakes can you make with 4 cups of flour?

Let x = amount of flour

y = number of cupcakes

Since y varies directly with x, y = kx

Find k when x = 1 and y = 6

6 = k × 1

6 = k

Therefore, y = 6x is the direct variation equation

When x = 4, y = 6 × 4 = 24. So you can make 24 cupcakes

Not every equation represents a direct variation or a direct proportion.

Examples of equations that are direct variations:

y = -4x

y = 5x

y = (-4/6)x

y = (2/3)x

y = 100x

Equations that are not

y = -4x + 2

y = 5x -5/7

y = (-4/6)x + 7

y = (2/3)x + 3

y = 100x + 5

Inverse variation