Distance rate time problems
Distance rate time problems involve object moving at a constant rate and this is called uniform motion. The formula d = r × t is the formula to use to solve problems related to distance, rate, and time.
Examples showing how to solve distance rate time problems
Case #1: Same direction travel
Let's say you and your wife are going to a place, but you each prefer to drive your own car.
It may be obvious that for this type of problem, the distance is the same since you left the same place (your house) and are going to the same location.
You leave your house at 7 AM and you drive 60 miles per hour. Your wife was taking her time. So she leaves the house at 9 AM. However, she is now speeding at 90 miles per hour.
How long will it take your wife to catch up with you assuming that the police do not stop her?
When your wife finally catches up with you, what distance have you both traveled?
It is always a good idea to make a table when solving distance rate time problems, especially if you are not familiar with distance word problems.
let t be the time it takes you to get to your destination.
t-2 is then the time it will take your wife. The reason we subtract 2 is because compared to you, your wife is late by 2 hours.
| Car | Rate | Time | Distance traveled |
| You | 60 | t | 60 × t |
| Your wife | 90 | t-2 | 90 × (t - 2) |
Since the distance you travel is the same as the distance your wife travels, we get:
60 × t = 90 × (t - 2)
60t = 90t - 180
60t + 180 = 90t
180 = 90t - 60t
180 = 30t
Since 30 times 6 = 180, t = 6
This means that your wife will catch up with you in 6 hours.
d = 60 × 6 = 360
d = 90 × (6-2) = 90 × 4 = 360
Therefore, your wife will catch up with you 360 miles away. Of course, this is assuming that you keep cruising at 60 miles per hour and she keeps cruising at 90 miles per hour.
Case #2: Round-trip travel
Let's say that you are going back to your house. When you get to your house, this is a round trip.
Suppose that you went to no other places, the distance then to go back to your house is again the same.
However, now you decide to speed things up a little bit. Instead of driving 60 miles per hour, you do 90 miles per hour.
If the round trip lasts 12 hours, how long does it take you to get back home?
let t be the time it takes you to get to your destination.
12 - t is then the time it will take you to get back home.
| Car | Rate | Time | Distance traveled |
| to your location | 60 | t | 60 × t |
| back to your house | 90 | 12 - t | 90 × (12 - t) |
Since the distance to go to your location and to go back home is the same, we get:
60 × t = 90 × (12 - t)
60t = 90 × 12 - 90t
60t = 1080 - 90t
60t + 90t = 1080 - 90t + 90t
150t = 1080
Since 150 times 7.2 = 1080, t = 7.2
12 - 7.2 = 4.8
Therefore, it took you 4.8 hours to get back home.
Case #3: Opposite direction travel
Suppose that you are driving south and your wife is going north on the same highway.
After a certain amount of time driving, the distance you travel will most likely be different than your wife's distance since it is not likely that your rate will be the same.
Therefore, when solving distance rate time problems involving opposite direction travel, you can add the distances to get the total distance.
Going south you drive 45 miles per hour. Going north your wife drives 30 miles per hour. After how long will you be 225 miles apart?
let t be the time it takes you to be 225 miles apart from your wife.
It will take your wife the same time to be 225 miles apart from you. I hope this makes sense!
| Car | Rate | Time | Distance traveled |
| You | 45 | t | 45 × t |
| Your wife | 30 | t | 30 × t |
The total distance you and your wife travel is 45t + 30t = 75t and this must be equal to 225
75 × t = 225
Since 75 times 3 = 225, t = 3
Therefore, you will be 225 miles apart after 3 hours.
The above are great examples of distance rate time problems. Try to do some now in your math textbook.
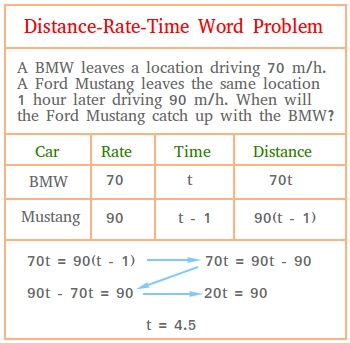
Study also the distance rate time problem in the figure below carefully