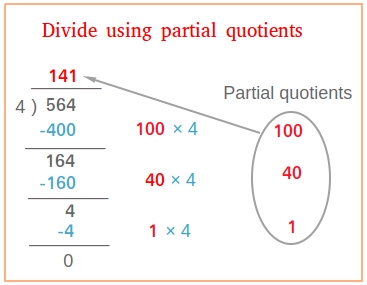
Divide using partial quotients
Divide using partial quotients is closely related to divide using repeated subtraction.
Examples showing how to divide using partial quotients
In the lesson about divide using repeated subtraction, we divided 28 by 5 and we came up with the following.
____
5 ) 28
-5 1
________
23
-5 1
_________
18
-5 1
__________
13
-5 1
__________
8
-5 1
__________
3
Notice that 5 is subtracted five times. Subtracting 5 five times is the same as subtracting 25. Therefore, instead of subtracting 5 five times, you can just subtract 25 once.
When you do it like that, you are just subtracting greater multiples of the divisor. Notice that we call 5 partial quotient. Although in this problem, there is only one partial quotient, you will in practice get more than 1 partial quotient as example #2 below shows.
____
5 ) 28 Partial quotients
-25 5 × 5 5
_________
3
Since 3 is less than 5, 3 is the remainder. Thus the answer is 5 r3
Example #2.
Use partial quotients to divide 496 by 4
Step 1
Subtract greater multiples of the divisor. Notice that if we were using repeated subtraction, we would have to subtract 4 one hundred times! Now you see why it is good to divide using partial quotients.
Step 2
Subtract lesser multiples of the divisor.
Step 3
Add the partial quotients. All the steps are shown below.
_______
4 ) 496 Partial quotients
-400 100 × 4 100
________
96
-80 20 × 4 20
________
16
-16 4 × 4 4
________
0
After adding the partial quotients, the answer is 100 + 20 + 4 = 124
496 ÷ 4 = 124
Example #3.
Use partial quotients to divide 786 by 7
_______
7 ) 786 Partial quotients
-700 100 × 7 100
________
86
-70 10 × 7 10
________
16
-14 2 × 7 2
________
2
After adding the partial quotients, we get is 100 + 10 + 2 = 112
We also have a leftover or a remainder of 2.
Therefore, using partial quotients, 786 divided by 7 is 112 r2
The meaning of 112 r2 is a quotient of 112 with a remainder of 2.
Did you find the examples above tough to understand? Did you not understand them at all? Please take a look at this figure!