Estimate the square root

One way to estimate the square root of any number, especially numbers that are not perfect squares, is to find a whole number greater than the square root and another whole number less than the square root of that number that is not a perfect square.

Take a close look at the figure below so you can learn the process. There are some important observations you need to make!

In our example below, since 34 is not a perfect square, we can try to find an approximation for the square root.

Perfect square numbers do not need estimation. You just need to know your multiplication table to find them. Many people know the perfect square numbers from 1 to 1000.

Notice that 34 is much closer to 36 than it is to 25. Therefore, we chose a number very close to 6 and that number is 5.8.

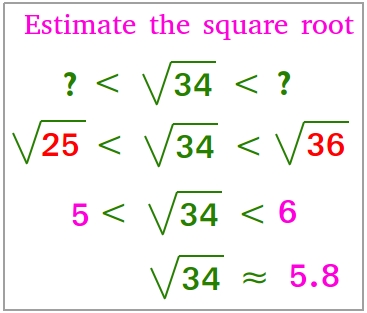
The example in the figure above is a quick and easy way to estimate a square root when it is not possible to find perfect square roots. You will learn two different methods in this lesson.

More examples showing how to quickly estimate the square root

We will illustrate the process with two more examples.

Example #1:

Estimate the square root of 17

We will find a whole number bigger than the square root of 17 and a whole number smaller than the square root of 17.

< √17 <

Study carefully the procedure!

First, let us find the number that is bigger than √17

√17 < √18

√17 < √19

√17 < √20

√17 < √21

√17 < √22

√17 < √23

√17 < √24

√17 < √25

Notice that the square roots of 18, 19, 20, 21, 22, 23, 24 are all bigger than the square root of 17.

However, only √25 will give a whole number, so this is the one we will choose.

√25 = 5 since 5 × 5 = 25

Second, let us find the number that is smaller than √17

√16 < √17

Since √16 is a whole number, this is the one we will choose.

√16 = 4 since 4 × 4 = 16

We get 4 < √17 < 5

The square root of 17 is between 4 and 5. We could estimate the square of 17 to be 4.1 for example.

Example #2:

Estimate the square root of 102

We will find a whole number bigger than the square root of 102 and a whole number smaller than the square root of 102.

√101   <   √102

√100   <   √102

We will use square root of 100 since √100   = 10

To find the number bigger than the square of 102, we will use a different strategy. It take too long to write down the square root of all these numbers. Some good observation will help us to solve the problem quickly.

Notice that the square root of any number between 103 and 120 is not a whole number.

However, square root of 121 is a whole number since 11 times 11 = 121.

Therefore, the square of 121 will give us the whole number that we need that is bigger than square of 102.

We get 10 < √102 < 11

The square root of 102 is between 10 and 11. We could estimate the square root of 102 to be 10.2.

Notice again that in our estimation, we chose a number close to 10 since 102 is much closer 100 than it is 121.

Another estimation method

Just study carefully the following steps outlined in example #3 and example #4. You will not get exact answers with this method, but it will help you get as close as possible.

Example #3

Estimate the square root of 45

Step 1

Pick a number you think √45 is close to. For example, pick 7 since √49 = 7. We did not pick 6 since √49 is closer to the answer than √36.

Step 2

Find the difference between the square of √49 and the square of √45

(√49)² - (√45)² = 49 - 45 = 4

Step 3

Divide the number you found in step 2 by twice the number you picked in step 1

4 / 2(7) = 4 / 14 = 0.2857

Step 4

Since 7 is an overestimation, subtract 0.2857 from 7 and this is your estimation.

7 - 0.2857 = 6.714

6.714 times 6.714 = 45.0777 and as you can see 45.0777 is quite close to 45

Example #4

Estimate the square root of 39

Step 1

Pick a number you think √39 is close to. For example, you can pick 6 since √36 = 6.

Step 2

Find the difference between the square of √39 and the square of √36

(√39)² - (√36)² = 39 - 36 = 3

Step 3

Divide the number you found in step 2 by twice the number you picked in step 1

3 / 2(6) = 3 / 12 = 0.25

Step 4

Since 6 is an underestimation, add 0.25 to 6 and this is your estimation.

6 + 0.25 = 6.25

6.25 times 6.25 = 39.0625 and as you can see 39.0625 is quite close to 39