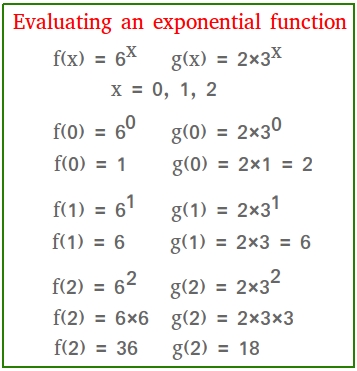
Evaluate an exponential function

This lesson will show how to evaluate an exponential function and how to solve a real-world problem by evaluating an exponential function.

An example showing how to evaluate an exponential function


Example #1

Evaluate the following function for x  = 0, 1, 2, -1, -2.

Notice that this time, we used a table to organize our calculations. It is always good practice to use a table when evaluating exponential functions.

f(x) = 4 × 5x

x
4 × 5x
 f(x)
0
4 × 50 = 4 × 1 = 4
 4
1
4 × 51 = 4 × 5 = 20
 20
2
4 × 52 = 4 × 5 × 5 = 4 × 25 = 100
 100
-1
4 × 5-1 = 4 × 1/5 = 4/1 × 1/5 = (4 × 1) / (4 × 1) = 4/5
 0.8
-2
4 × 5-2 = 4 × 1/25 = 4/1 × 1/25 = (4 × 1)/ (1 × 25) = 4/25
 0.16

Notice that you can also write the answers as shown below:

f(0) = 4

f(1) = 20

f(2) = 100

f(-1) = 0.8

f(-2) = 0.16

Evaluate an exponential function to solve a real-world problem.


Example #2

Suppose 30 toads are taken to an island. The toad population quintuple every 3 months. Model this situation with an exponential function and then evaluate the function to find how many toads would be there after 4 years.

Solution

There are two Important keys words to understand in this problem and these are 'quintuple' and 'every 3 months'.

Quintuple:

The word quintuple means that the population was increased 5 times.

Every 3 months:

Every 3 months is the same as every one-fourth of a year or 1 quarter.

Let x be the number of quarters, then f(x) = 30 × 5x

For this problem, there are 4 quarters in a year since the growth is happening every 3 months. For two years then, we get 8 quarters.

f(8) = 30 × 58

f(8) = 30 × 390625

f(8) = 11718750

After two years, there will 11,718,750 toads in that island.

Notice that when x = 0, f(0) = 30×50

f(0) = 30×1 
f(0) = 30.

x = 0 refers to the starting point or the day the toads were taken to the island. 

A little summary on how to evaluate an exponential function

Evaluating an exponential function

Exponential and logarithmic functions

How to evaluate logarithms