Expanding logarithms

The examples below will teach you about expanding logarithms using the properties of logarithms, also called rules of logarithms. First study the example in the figure below carefully so that you can understand the process clearly.

Notice that after expanding log_b[(x²y) / z] we get the expression below shown in blue.

2log_b x + log_b y - log_b z

Notice also that expanding logarithms is the inverse of simplifying logarithms. You are trying to express a single logarithm into many individual logarithms.

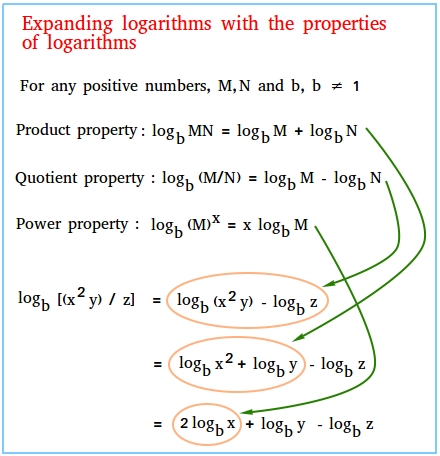
How to use the rules of logarithms to expand a logarithmic expression

Let us see how we expanded the logarithmic expression log_b[(x²y) / z] step by step.

To expand log_b[(x²y) / z] you need to use a combination of the product property, the quotient property, and the power property.

First, use the quotient rule which says that the logarithm of a quotient is equal to the difference of the logarithms.

log_b (M/N) = log_b M - log_b N

log_b[(x²y) / z] = log_b x²y - log_b z

Second, use the product rule which says that the logarithm of a product is equal to the sum of the logarithms.

log_b (MN) = log_b M + log_b N

Use the product rule to rewrite log_b x²y.

log_b[(x²y) / z] = log_b x² + log_b y - log_b z

Lastly, use the power rule which says that the logarithm of a power is equal to the exponent multiplied by the logarithm of the base of the exponential number.

logb (M)^x = xlog_b M

Use the power rule to rewrite logb x².

log_b[(x²y) / z] = 2log_b x + logb y - logb z

More examples showing how expanding logarithms work

Example #1

Expand log₅ (3xy)

In this example, you just need to use the product property.

log₅ (3xy) = log₅ 3 + log₅ x + log₅ y

Example #2

Expand log (a²b³)

In this example, you need to use a combination of the product property and the power property.

log₁₀ (a²b³) = log₁₀ a² + log₁₀ b³

= 2log₁₀ a + 2log₁₀

Example #3

Expand log₂ (5x / 10y)

In this example you need to use a combination of the product property and the quotient property.

log₂ (5x / 10y) = log₂ (5x) - log₂ (10y)

= log₂ (5) + log₂ (x) - [ log₂ (10) + log₂ (y) ]

= log₂ (5) + log₂ (x) - log₂ (10) - log₂ (y)

= log₂ (5) + log₂ (x) - log₂ (5×2) - log₂ (y)

= log₂ (5) + log₂ (x) - (log₂ 5 + log₂ 2) - log₂ (y)

= log₂ (5) + log₂ (x) - log₂ 5 - log₂ 2 - log₂ (y)

= log₂ (x) - log₂ 2 - log₂ (y)

Notice that log₂ (5) - log₂ 5 = 0

Notice also that if you remember to simplify a quotient, there will be less work to do as demonstrated below for example #3

log₂ (5x / 10y) = log₂ (x / 2y)

= log₂ x - log₂ 2y

= log₂ x - ( log₂ 2 + log₂ y )

= log₂ x - log₂ 2 - log₂ y

How to expand the logarithm of a radical expression

To expand the logarithm of a radical expression, begin by rewriting the radical expression as a fractional exponent. Once you have done that, continue by applying the rules of logarithms.

Example #4

Expand log₃ [ √ (36x³y) ]

Using a combination of the product property and the power property,

log₃ [ √ (36x³y) ] = log₃ [ (36x³y)^1/2 ]

= 1/2 log₃ (36x³y)

= 1/2 [ log₃ (36) + log₃ (x³) + log₃ (y) ]

= 1/2 [ log₃ (36) + 3log₃ (x) + log₃ (y) ]

= 1/2 log₃ (36) + 3/2 log₃ (x) + 1/2 log₃ (y)

Notice that if you are trying to expand the natural logarithm of an expression, you can just use the rules mentioned in the figure above.

Example #5

Express ln 8x²y³ as a sum of 3 natural logarithms.

Use the product rule

ln 8x²y³ = ln 8 + ln x² + ln y³

Use the power rule