What is Factoring in Math? Definition and Examples

Factoring is a fundamental skill in algebra that involves rewriting mathematical expressions as products of their factors. By factoring, you essentially reverse the multiplication process, breaking down complex expressions into simpler, more manageable parts. This skill is crucial for solving equations and simplifying expressions.

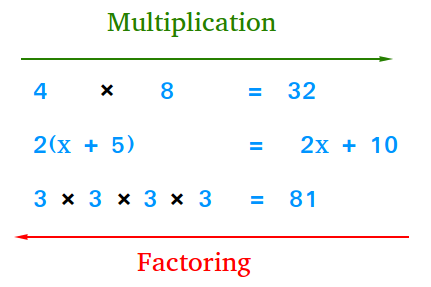
Examples of Factoring:

Simple Numerical Factoring:

Example: 32 = 4 × 8

Explanation: By referring to the multiplication table, it's easy to identify that 4 and 8 are factors of 32

Prime Factorization:

Example: 81 = 3 × 3 × 3 × 3

Explanation: Breaking down a number into its prime factors helps in understanding its fundamental composition.

Factoring Out the Greatest Common Factor (GCF):

Example: 2x + 10 = 2(x + 5)

Explanation: Here, 2 is the GCF of the terms 2x and 10

Factoring Complex Algebraic Expressions:

Examples:

• x² - 14x - 32
• 15x² - 26x + 11
• 150x³ + 350x² + 180x + 420

Explanation: These expressions require more advanced factoring techniques to break them down into simpler binomial or trinomial factors.

Prerequisites for Factoring

Both numerical and algebraic expressions can be factored using some specific method(s). 
The simplest expressions to factor are of course numerical expressions. However, looking for the prime factorization of a big number like 240 or an expression such as 150x³ + 350x² + 180x + 420 may require a lot more work. 

Before diving into factoring various types of expressions, it's essential to have a solid understanding of the following concepts:

• Factoring Integers: Understanding how to break down numbers into their constituent factors is crucial for more complex factoring tasks.

• Finding the Greatest Common Factor (GCF): Identifying the GCF of terms is a foundational step, especially when factoring polynomials or expressions with multiple terms.

• Multiplying Binomials: Familiarity with binomial multiplication is necessary for recognizing patterns and reversing the multiplication process during factoring.

Types of Factoring

Factoring can be categorized into several types, each suitable for different kinds of expressions. Below are the primary methods of factoring algebraic expressions:

1. Factoring by Finding the Greatest Common Factor (GCF)

Description: Identify and factor out the largest common factor from all terms in the expression.

Example: 6x³+9x² = 3x²(2x+3)

GCF: 3x²

2. Factoring Trinomials

Description: Factor expressions of the form x²+bx+c by finding two binomials that multiply to give the original trinomial.

Example: x²+5x+6=(x+2)(x+3)

Methods for Factoring Trinomials:

• Standard Method: Look for two numbers that multiply to c and add to b.
• Box Method: Organize terms in a grid to systematically find factors.
• Factoring by Grouping: Group terms to find common factors within each group.
• Removing the Impostor : Adjust the trinomial to facilitate easier factoring by eliminating conflicting terms.

3. Factoring Perfect Square Trinomials

Description: Recognize and factor trinomials that are perfect squares, i.e., expressions that are squares of binomials.

Example: x²+6x+9=(x+3)²

4. Factoring Using the Quadratic Formula

Description: Apply the quadratic formula to find the roots of the quadratic equation and express the trinomial as a product of binomials.

Quadratic Formula: x =[(−b± √(b2−4ac))] / 2a

Example: For x²−5x+6

• Roots: x=2 and x=3
• Factored Form: (x−2)(x−3)

5. Factoring by Grouping

Description: Group terms with common factors and factor each group separately before combining them.

Example: ax+ay+bx+by = a(x+y) + b(x + y) = (x+y)(a+b)