Graphing exponential growth
When graphing exponential growth, it is always a good idea to make a table of values and you could look for at least 5 points that you can use to make the graph.
It is also a good idea to choose both negative and positive values for x, especially numbers that are opposites. For example, notice how we chose x = -2 and x = 2.
| x | ||
| -2 | ||
| -1 | ||
| 0 | ||
| 1 | ||
| 2 |
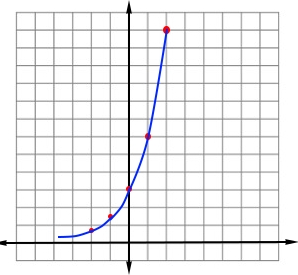
Did you make the following observations about the figure above?
- Each square in vertical axis or y-axis represents 1 unit.
- Each square in horizontal axis or x-axis represents 1 unit.
Important observation when graphing exponential growth
Now, let us see what will happen if we choose the following values for x:
x = -4, -10, -20
If x = -4, y = 3 × 2-4 = 3 / 24 = 3 / 16 = 0.1875.
If x = -10, y = 3 × 2-10 = 3 / 210 = 3 / 1024 = 0.00292968.
If x = -20, y = 3 × 2-20 = 3 / 220 = 3 / 1048573 = 0.00000286.
As the values of x get smaller and smaller, the values of y get smaller and smaller as well. Notice that these the values of y will get closer and closer to 0.
Now, let us see what will happen if we choose the following values for x:
x = 4, 10, 20
If x = 4, y = 3 × 24 = 3 × 24 = 3 × 16 = 48.
If x = 10, y = 3 × 210 = 3 × 210 = 3 × 1024 = 3072.
If x = 20, y = 3 × 220 = 3 × 220 = 3 × 1048576 = 3145728.
As the values of x get bigger and bigger, the values of y get bigger and bigger as well. Notice that these the values of y will approach infinity.