How to simplify logarithms
Find out how to simplify logarithms by writing a logarithmic expression as a single logarithm with these exercises.
Examples showing how to simplify logarithms
Exercise #1
Simplify log3 40 - log3 10
Using the quotient property, log3 40 - log3 10 = log3 40 / 10
Simplify log3 40 / 10 to get log3 4
log3 40 - log3 10 = log3 4
Exercise #2
Simplify log4 3 + log4 6
Using the product property, log4 3 + log4 6 = log4 3 x 6
Simplify log4 3 x 6 to get log4 18
log4 3 + log4 6 = log4 18
Exercise #3
Simplify log10 9 + log10 5 - log10 15
Using a combination of the product rule and the quotient rule, we can simplify this logarithmic expression as shown below.
log10 9 + log10 5 - log10 15 = log10 ( 9 x 5 ) - log10 15
= log10 ( 45 ) - log10 15
= log10 ( 45 / 15 )
= log10 3
Exercise #4
Simplify log5 1 / 8 + 3 log5 4
Using a combination of the product rule and the power rule, we can simplify as shown below.
log5 1 / 8 + 3 log5 4 = log5 1 / 8 + log5 43
= log5 1 / 8 + log5 64
= log5 (1 / 8 x 64 )
= log5 ( 64 / 8 )
= log5 8
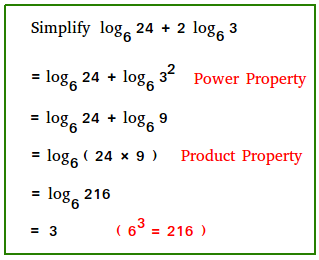
Here is yet another example clearly showing how to simplify a logarithmic expression using the properties of logarithms.
In the example below, we use the power property and the product property to simplify log6 24 + 2 log6 3. Logarithms can be simplified using only one property or a combination of all 3 properties.
Recent Articles
-
45-45-90 Triangle
May 01, 23 07:00 AM
What is a 45-45-90 triangle? Definition, proof, area, and easy to follow real-world examples. -
Theoretical Probability - Definition, Explanation, and Examples
Apr 24, 23 07:02 AM
Learn how to compute the likelihood or probability of an event using the theoretical probability formula.
100 Tough Algebra Word Problems.
If you can solve these problems with no help, you must be a genius!
