Logarithm of a negative number?

We will show that the logarithm of a negative number or zero is undefined or does not exist.

Similarly, you cannot find the logarithm of the following expressions.

log₅ -125     

log₁₀ -100                    

log₅ 0                

log₄ 0

Why the logarithm of a negative number cannot be negative?

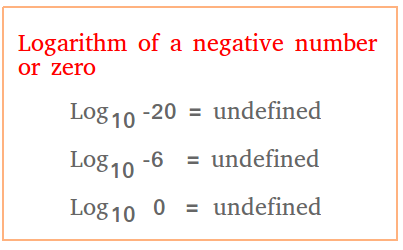
The reason for this is that any positive number b raised to any power x cannot equal to a number y less than or equal to zero.

The definition says If y = b^x, then log_b y = x

If x is bigger than zero or x is equal to zero, It is obvious that b^x will be bigger than zero as you can see in the examples below. As a result, y will also be bigger than zero since y = b^x

65⁰ = 1

7⁰  = 1

24¹ = 24

2³ = 8

4² = 16

5³ = 125

6⁴  =1296

8⁵ = 32768

How about when x is negative?  

Let x  = -2, -3, and -8 and let b = 5

y = 5^-2 = 1 / 5² = 1 / 25 = 0.04

y = 5^-3 = 1 / 5³ = 1 / 125 = 0.008

y = 5^-8 = 1 / 5⁸ = 1 / 390625 = 0.00000256

Since y can never be zero or negative, it does not make sense to replace y in
log_b y with zero or a negative number.

Now, you can clearly see why these expressions do not make sense

log₅ -125                       log₁₀ -100                     log₅ 0                 log₄ 0

In fact, for log₅ -125, there is no number x, such 5^x = -125

If you choose 3, you will get 5³ = 125 and if you choose -3, you will get 5^-3 = 0.008

If it did not work for x = 3 and x = -3, no other numbers will work!

By the same token, for log₅ 0, there is no number x such that 5^x = 0