Measures of Dispersion
Measures of dispersion, also called measures of variation, are measures that help us to know about the spread or variation of a data set.
At this point, you may not quite understand what we mean by spread or variation. We will illustrate with an example.
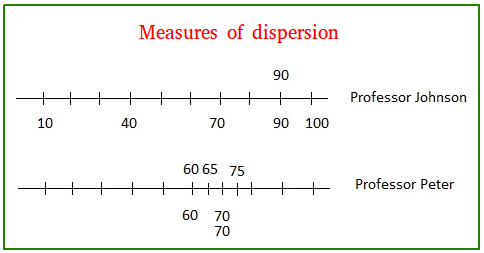
Suppose that "professor Johnson" teaches calculus 1 and "professor Peter" teaches also calculus 1.
After some research was done, the following was discovered:
The grades of 6 students chosen randomly attending Johnson's class tend to be
100 10 70 90 40 90
The grades of 6 students chosen randomly attending Peter's class tend to be
70 60 70 65 60 75
Put the grades on a number line as seen in the figure above. Looking at the number line, what can you conclude about the classes?
You may find it surprising that the average grade is the same for both classes.
Indeed, the average grade for both classes is 66.66.
What else can we conclude from the graph?
The performance of the students in Peter's class is almost the same.
Since the grades are close together, we say that the grades in Peter's class have a low measure of dispersion or a low measure of variation.
However, the grades in Johnson's class are spread farther apart. Therefore, the measure of dispersion is larger.
How to calculate the measures of dispersion
How big though is the dispersion or variation? We can use the range to find it.
The range is the simplest measure of dispersion to calculate.
To find it, use the following formula
Range = Highest value - Smallest value
The range for Johnson's class is 100 - 10 = 90
The range for Peter's class is 75 - 60 = 15
You may even be able to make some conclusions on the teaching style of the professors or on the way the students behave in class.
If we are trying to blame it on professor Johnson, you could make the following conclusions:
- May be professor Johnson does not reach out to help everyone in the class.
- May be his teaching style does not work for everyone.
- May be he does not encourage group work.
The grades for Peter's class are not that great. However, it is possible that whatever the students get, they all pretty much get it at the same time.
Since the range is very affected by extreme values, it is not a very reliable measure of variation.
Next lesson, you will learn about the standard deviation, which is the most important and probably the most accurate measure of dispersion.