Multiplying complex numbers
When multiplying complex numbers, many properties of real numbers such as the distributive property and/or FOIL, are also valid for complex numbers.
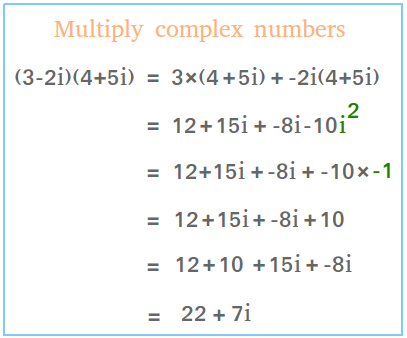
The multiplication of complex numbers is basically the same as the multiplication of polynomials as you can see in the example above.
After completing the multiplication, just replace any occurrences of i2 with -1 and then simplify by adding the real parts together and the imaginary parts together.
In the example below, the real parts are 12 and 10 and the imaginary parts are 15i and -8i. If you did not understand the example below, keep reading as we explain how to multiply complex numbers starting with the easiest examples and moving along with more complicated ones.
More examples about multiplying complex numbers.
Example #1:
Multiply 6 by 2i
6 × 2i = 12i
Example #2:
Multiply 5i by -3i
5i × -3i = -15i2
= -15(-1) Substitute -1 for i2
= 15
Example #3:
Multiply 5i by (-2i + 1)
5i × (-2i + 1) = 5i × -2i + 5i × 1 (distributive property)
= -10i2 + 5i
= -10(-1) + 5i
= 10 + 5i
Example #4:
Multiply (-2i + -3) by (-5i + 6)
(-2i + -3) × (-5i + 6) = -2i × (-5i + 6) + -3 × (-5i + 6)
(-2i + -3) × (-5i + 6) = -2i × -5i + -2i × 6 + -3 × -5i + -3 × 6
= 10i2 + -12i + 15i + -18
= 10(-1) + 3i - 18
= -10 + 3i - 18
= -28 + 3i
Example #5:
Multiply (3i + 4) by (3i - 4)
(3i + 4) × (3i - 4) = 3i × (3i - 4) + 4 × (3i + -4)
(3i + 4) × (3i - 4) = 3i × 3i + 3i × -4 + 4 × 3i + 4 × -4
(3i + 4) × (3i - 4) = 9i2 + -12i + 12i + -16
(3i + 4) × (3i - 4) = 9(-1) + 0 + -16
(3i + 4) × (3i - 4) = -9 + 0 + -16
(3i + 4) × (3i - 4) = -9 + -16
(3i + 4) × (3i - 4) = -25
Notice that you could have found the answer a little faster if you used this formula: (a - b)(a + b) = a2 - b2
(3i + 4) × (3i - 4) = (3i)2 - 42
= 9i2 - 16
= 9(-1) - 16
= -9 - 16
= -25