Percentage word problems
Before you take a look at the percentage word problems in this lesson and their solutions, it may help to review the lesson about formula for percentage or you can use the different techniques that I use here.
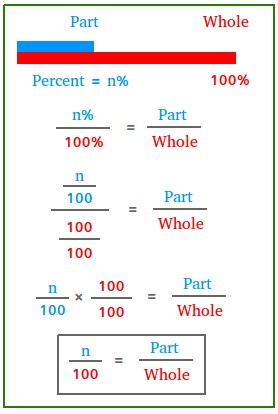
Different types of percentage word problems
There are three different types of percentage word problems. We will show how to solve them using proportions.
- What is 80% of 20? (example #1)
- 50 is 25% of what number? (example #2)
- 18 is what percent of 24? What percent of 2000 is 3500? (example #3 and example #4)
Solving percentage word problems using proportions
You can solve problems involving percents using the proportion you see in the figure above:
(n% / 100% = Part / Whole)
First, study the figure carefully! Then, we will show how to use the proportion to solve percentage word problems by creating diagrams to visualize relationships.
Example #1:
A test has 20 questions. If peter gets 80% correct, how many questions did peter miss?
Solution
First, you need to find the number of correct answers by looking for 80% of 20.
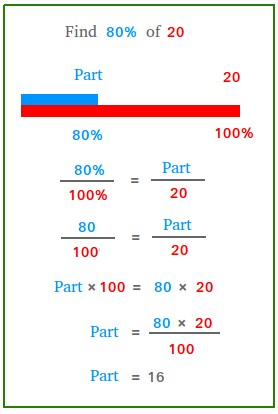
When the problem involves looking for the part or the problem says something like, "Find 80% of 20" or "Find 30% of 50," just change the percent to a decimal and multiply.
80% of 20 = (80 / 100) × 20 = 0.80 × 20 = 16
Since the test has 20 questions and he got 16 correct answers, the number of questions Peter missed is 20 − 16 = 4
Recall that 16 is called the percentage. It is the answer you get when you take the percent of a number.
Percentage = Part
Example #2:
In a school, 25% of the teachers teach basic math. If there are 50 basic math teachers, how many teachers are there in the school?
Method #1
Once again set the problem up as shown in the figure below. Notice that the question is, " How many teachers are in the school?"
Therefore, the whole is missing this time!

Method #2
I shall help you reason the problem out!
When we say that 25% of the teachers teach basic math, we mean 25% of all teachers in the school equals number of teachers teaching basic math.
Since we don't know how many teachers there are in the school, we replace this with x or a blank.
However, we know that the number of teachers teaching basic math is equal to the percentage = part = 50
Putting it all together, we get the following equation:
25% of ____ = 50 or 25% × ____ = 50 or 0.25 × ____ = 50
Thus, the question is 0.25 times what gives me 50?
A simple division of 50 by 0.25 will get you the answer
50 / 0.25 = 200
Therefore, we have 200 teachers in the school
In fact, 0.25 × 200 = 50
More percentage word problems
Example #3:
24 students in a class took an algebra test. If 18 students passed the test, what percent do not pass?
Solution
First, find out how many student did not pass.
Number of students who did not pass is 24 − 18 = 6
Then, write down the following equation:
x% of 24 = 6 or x% × 24 = 6
To get x%, just divide 6 by 24
6 / 24 = 0.25 = 25 / 100 = 25%
Therefore, 25% of students did not pass.
Example #4:
A fundraising company would like to raise $2000 for a cause. The fundraiser was so successful that they ended up raising $3500. What percent of their goal did they raise?
Notice that the whole is 2000 since this is the whole money they expect to raise. The part is the amount that the fundraiser ended with and it usually lower than the amount they expect to raise. However, in this particular case, the part ended up being bigger than the whole. Keeping this in mind, here is how to set it up and solve it!
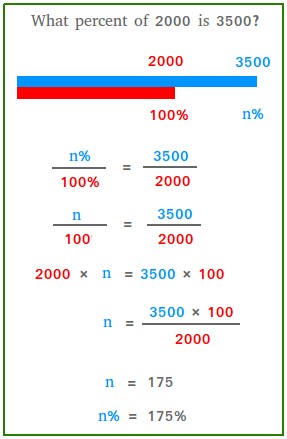
The fundraising company was able to raise 175% of the expected amount.
Example #5:
A department has a total of 22,000 units of stock. 25% of the garments are black and 10% of the garments are size 14.
a) How many black garments are there?
b) How many size 14 garments are there?
c) If 10% of the black garments are size 14,how many garments are black and size 14?
Note that the solution we show below for example #5 use a completely different approach or technique. Read it carefully and try to learn it as well!
Solution
25% = 25 per 100 = 250 per 1000
For 22,000 just multiply 250 by 22
250 × 22 = 250 × (10 + 10 + 2)
= 2500 + 2500 + 500
= 5000 + 500
= 5500
So, there are 5500 black garments.
10% = 10 per 100 = 100 per 1000
For 22,000 just multiply 100 by 22
100 × 22 = 2200
So, 2200 of the garments are size 14.
If 10% or 10 per 100 of the black garments are size 14, then 100 per 1000 of the black garments are size 14.
500 per 5000 are size 14. However, you need to find it for 5500 black garments.
Then, what is 10% of 500?
10% = 10 per 100, so 50 per 500.
So 550 of the black garments are size 14.
If you really understand the percentage word problems above, you can solve any other similar percentage word problems. If you still do not understand them, I strongly encourage you to study them again and again until you get it.
The end result will be very rewarding!