Permutations

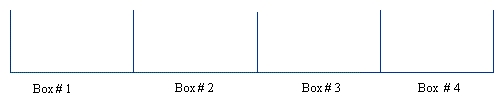
Permutations are ordered arrangement of objects. We have some good illustrations for you here! For example, there are 24 ways to arrange or put 4 books in 4 boxes as shown in the figure below:

Objects stand for anything you are trying to arrange or put in a certain order.

Other examples of arrangements are:

• You have 5 CDs. How many different ways can you listen to them?

• You are seating 3 people on 3 chairs. How many different ways can people sit?

• You have 6 books to read. How many different ways can you read your books?

Before solving the situations above, let us make sure you understand how many different ways there are to arrange 4 books in 4 boxes. 

Since you have 4 boxes, you have 4 choices to put the first book. You can put the first book in any of those boxes. The important thing is to see that you have 4 choices to do this.

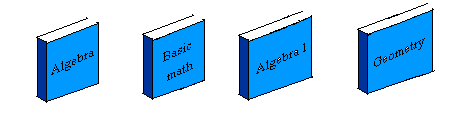
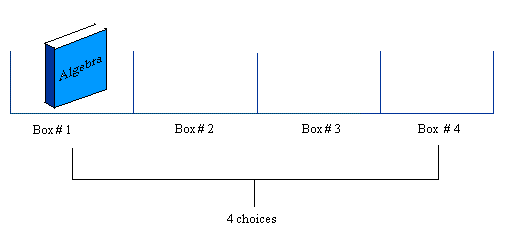
Say you put algebra in box #1

After you put the algebra book in box #1, you can no longer use box #1. You can use box #2, box #3, or box #4 to place your next book.

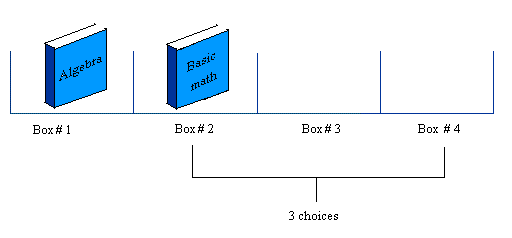
This means you have 3 choices to place your next book. Say that you put basic math in box #2

After you put the basic math book in box #2, you can no longer use box #2. You can use box #3 or box #4 to place your next book.

Say that you put the algebra 1 book in box #3

After you put the algebra 1 book in box #3, you have only 1 choice ( box #4) to place your last book, which is geometry

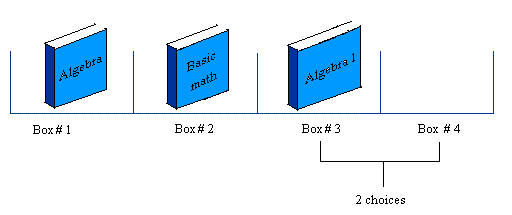
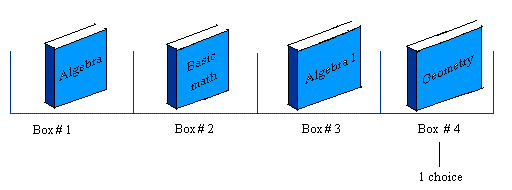
Now to find the total number of arrangement, you need to multiply all your choices as seen in the fundamental counting principle.

Therefore,

Number of permutations = 4 × 3 × 2 × 1 = 24

More examples showing how permutations work

Let us now solve the situations above.

How many ways can 3 people sit on 3 chairs?

The first person has 3 choices

Once the first person sits, there are only 2 seats left.

Thus, the second person who sits has 2 choices

The last person has 1 choice

The number of ways people can sit = 3 × 2 × 1 = 6 ways


How many different ways can you listen to five CDs?

Assuming you do not listen twice to a CD, you have 5 choices to listen to the first CD.

4 choices to listen to the second CD

3 choices to listen to the third CD

2 choices to listen to the fifth CD

1 choice for the last CD

So you have 5 × 4 × 3 × 2 × 1 = 120 different ways to listen to the 5 CDs