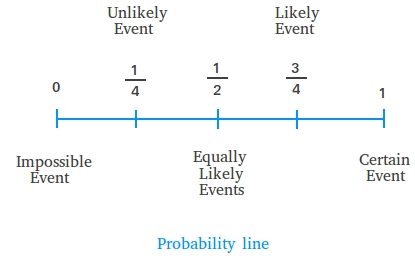
Probability line

The probability line is a line that shows probabilities and how these probabilities relate to each other.

Since the probability of an event is a number from 0 to 1, we can use the probability line below to show the possible ranges of probability values.

The line shows that if an event is certain or sure to happen, it will have a probability of 1.

For example, the probability that it will rain in the state of Florida at least once in a specific year is 1.

The number line shows that if an event will never happen or cannot happen, it will have a probability of 0.

For example, the probability that you can pick a red ball from a bag containing 4 green balls and 5 blue balls is 0.

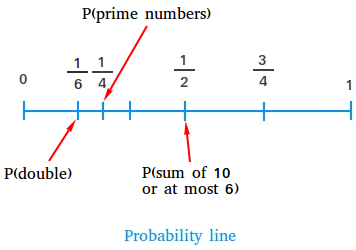
Showing probability on a probability line with an example.

If you throw a pair of dice, you can use a number line to show the probabilities of getting a double, prime numbers only, numbers whose sum is ten or at the most six.

First, we need the sample space when we throw two dice.

(1,1), (1,2), (1,3), (1,4), (1,5), (1,6), (2,1), (2,2), (2,3), (2,4), (2,5), (2,6), (3,1), (3,2), (3,3), (3,4), (3,5), (3,6), (4,1), (4,2), (4,3), (4,4), (4,5), (4,6), (5,1), (5,2), (5,3), (5,4), (5,5), (5,6), (6,1), (6,2), (6,3), (6,4), (6,5), and (6,6)

What is the probability of getting a double?

The doubles are (1,1),(2,2), (3,3),(4,4),(5,5), and (6,6). There are 6 doubles and 36 possibilities in the sample space then.

The probability to get a double is 6/36 = 1/6

What is the probability of getting prime numbers only?

The prime numbers are (2,2), (2,3), (2,5), (3,2), (3,3), (3,5), (5,2), (5,3), and (5,5). There are 9 possibilities to get prime numbers only and 36 possibilities in the sample space then.

The probability to get prime numbers only is 9/36 = 1/4

What is the probability of getting numbers whose sum is ten or at the most 6?

Pairs of dice that give a sum of ten are (4,6), (5,5), and (6,4) 

Pairs of dice whose sum is at most six are (1,5), (2,4), (3,3), (4,2), (5,1), (1,4), (2,3), (4,1), (3,2), (1,3), (2,2), (3,1), (1, 2), (2,1), and (1,1) 

There are 3 possibilities to get pairs of dice whose sum is ten and 15 possibilities to get pairs of dice whose sum is at most six. 

The probability to get either a sum of at most 6 or 10 is 18/36 = 1/2

The probabilities are shown on the following probability line.