Properties of logarithms

The three main properties of logarithms are the product property, the quotient property, and the power property.

The table below will help you understand the properties of logarithms quickly.

Regarding the table that shows the logarithm of x for different values of x, notice that log x  = log10 x 

If you do not see the base next to log, it always means that the base is 10. We have also rounded to the nearest thousandth.

Properties of logarithms
x 1 2 3 4 5 6 7 8 9 10 15 20
log x 0 0.301 0.477 0.602 0.699 0.778 0.845 0.903 0.954 1 1.176 1.301


Now, what do you notice about the following pairs of statements?

log 2 + log 3 and log (2 x 3)

log 4 + log 5 and log (4 x 5)

Here is what we notice about them.

log 2 + log 3 = 0.301 + 0.477 = 0.778 and 0.778 = log (6) = log (2 x 3)

Therefore, log 2 + log 3 = log (2 x 3)

By the same token,

log 4 + log 5 = 0.602 + 0.699 = 1.301 and 1.301 = log (20) = log (4 x 5)

Therefore, log 4 + log 5 = log (4 x 5)

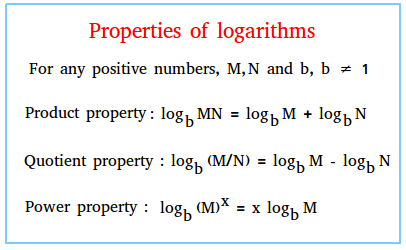
In general, for any positive numbers, M, N and b, where b is not equal to 1, we have the following product property

logb MN = logb M + logb N

Second, what do you notice about the following pairs of statements?

log (10 / 5) and log 10 - log 5

log (8 / 2)  and log 8 - log 2

Here is what we notice about them.

log 8 - log 2 = 0.903 - 0.301 = 0.602 and 0.602 = log (4) = log (8 / 2)

Therefore, log 8 - log 2 = log (8 / 2)

By the same token,

log 10 - log 5 = 1 - 0.699 = 0.301 and 0.301 = log (2) = log (10 / 5)

Therefore, log 10 - log 5 = log (10 / 5)

In general, for any positive numbers, M, N and b, where b is not equal to 1, we have the following quotient property

logb (M / N) = logb M - logb N

Finally, what do you notice about the following pairs of statements?

log (23) and 3 x log 2

log (32) and 2 x log 3

Here is what we notice about them.

log (23) = log 8 = 0.903

3 x log 2 = 3 x 0.301 = 0.903

Therefore, log (23) = 3 x log 2

log (32) = log 9 = 0.954

2 x log 3 = 3 x 0.477 = 0.954

log (32) = 2 x log 3

In general, for any positive numbers, M, N and b, where b is not equal to 1, we have the following power property

logb (Mx) = x logb M

Other properties of logarithms

Equality property: 

If logb M = logb N, then M = N

Change of base property: 

logb M = (loga M) / loga b 

Others

logb b = 1

logb 1 = 0

logb bM = M

What is a logarithm?