Quartiles and interquartile range

Quartiles and interquartile range are among the measures of position. Just like the name indicates, quartiles divide a ranked data (ordered set of data ) into four equal parts. Only three measures are needed to divide any data set into four equal parts.

These three measures that will divide an ordered data set into four equal parts are the first quartile (denoted by Q₁), the second quartile (denoted by Q₂), and the third quartile (denoted by Q₃).

After a data set has been ordered, or written in increasing order, here is how we define the quartiles.

The second quartile or Q₂  is the same as the median of the data set after the set has been ordered.

The first quartile or Q₁ is the median between the smallest number and the second quartile.

The third quartile or Q₃ is the median between the second quartile and the biggest number.

Now, take a look at the following figure and then make the following important observations.

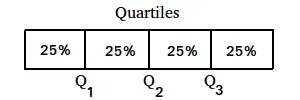
• About 25% of the values in the ranked data set are less than Q₁ 

• You can also say that about 75% of the values in the ranked data set are greater than Q₁

• About 75% of the values in the ranked data set are less than Q₃

• You could also say that about 25% of the values in the ranked data set are greater than Q₃

• About 50% of the values in the ranked data set are smaller than  Q₂ and about 50% are greater than  Q₂

Interquartile range

The interquartile range is the difference between third quartile and the first quartile.

IQR = Interquartile range = Q₃ - Q₁