Rational Numbers
Rational numbers are any numbers that can be written as a fraction. In other words, you can rewrite the number so it will have a numerator and a denominator.
They have the form
a
b
in which a and b are integers and b not equal to zero.
Notice that we said b cannot be zero. It is because any number divided by 0 has no answer.
Examples of rational numbers
|
2
3
|
,
5
2
,
|
1
4
, 2 ,
|
-8
2
, and 0
|
|
2
3
|
,
5
2
,
|
1
4
, 2 ,
|
-8
2
, and 0
|
Now, why are 2 and 0 rational numbers as well?
It is because 2 and 0 can be written as
|
2
1
|
and
0
x
|
|
2
1
|
and
0
x
|
x can be any number since 0 divided by any number is zero. Notice though that according to the definition x cannot be zero!
Decimal expansion of rational numbers
We can also write rational numbers as decimals.We do this by performing a quick division. We divide the numerator by the denominator.
For instance,
2
5
= 0.4
2
5
= 0.400000000
Notice that you can continue division to keep getting zeros for the decimal places after 4.
The bar on top of 0 means that if we continue to perform long division, we will keep getting an infinite number of zeros.
Another way to convert
2
5
into a decimal is to notice that we can multiply 5 by 20 and 2 by 20
We will get
40
100
And
40
100
= 0.40
Dividing by 100 or any other power of 10 is a straightforward process.
If you are dividing by 10, just move the decimal point one place to the left.
If you are dividing by 100, just move the decimal point 2 places to the left.
and so forth...
For
40
100
, the decimal point is after 0 for 40.
Moving that two places to the left bring the decimal point right before the 4.
A rational number can have either repeating decimal expansion or terminating decimal expansion.
Repeating decimal expansion:
A decimal expansion in which the numbers repeat in exactly the same order.
For example, 0.251251251251 is a repeating decimal expansion because 251 keeps repeating in the same order.
Terminating decimal expansion:
A decimal expansion that ends in all zeros.
For example, 0.150 is a terminating decimal expansion.
Here is a figure summarizing rational numbers or the "different types of rational numbers"
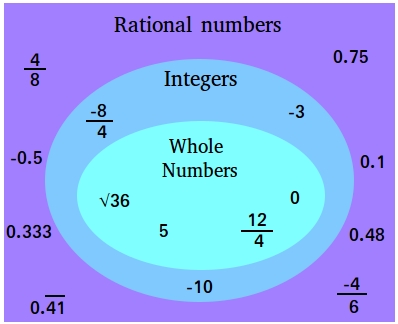
- Natural numbers are rational numbers since any natural number can be written in the form a/b. For example, 2 can be written as 2/1.
- Whole numbers are rational numbers since any whole number can be written in the form a/b. For example, 9 can be written as 9/1.
- Integers are rational numbers since any integer can be written in the form a/b. For example, -8 can be written as -8/1.
- Non-terminating decimal numbers with repeating patterns are rational numbers. For example, 0.2121212121 is a rational number since it can be written as 7/33.
- Terminating decimal numbers are rational numbers. For example, 0.75 is a rational number since it can be written as 3/4.
- Simple fractions whose numerators and denominators are whole numbers are rational numbers. For example, the fraction 2/5 is a rational number. However, the fraction 1.3/5 is not a rational number since 1.3 is not an integer according to the definition of a rational number.
Rational numbers FAQs
-
No. 0.6666666 is a rational number. However, it is not an integer. Some rational numbers are integers. For example, -8/1, 5/1 are rational numbers and integers as well since -8/1 = -8 and 5/1 = 5. Nonetheless, every integer is a rational number!
-
Yes, rational numbers are real numbers since real numbers include all sets of numbers except complex numbers.
-
No, not necessarily!There are negative rational numbers and positive rational numbers such as -1/2, 15/6, -4/3, 6/20.
-
3.14 is a famous irrational number known as pi. 3.14 has an infinite number of digits after the decimal point that do not have repeating patterns. Therefore, pi cannot be written as a ratio or be a rational number.
-
As a rule of thumb, if the number has a bunch of different digits after the decimal point with no specific pattern, the number is not rational.