Solve logarithmic equations

This lesson will discuss two ways to solve logarithmic equations. The method you use depends on the type of logarithmic equation you are trying to solve. Keep reading and you will learn how to solve the one below in a heartbeat!

The first type you will learn to solve has the following format

log_b X = Y

To solve it, you just have to write it in exponential form as X = b^Y

Example #1

log (2x + 1) = 3

log₁₀ (2x + 1) = 3

After writing it in exponential form we get

2x + 1 = 10³

2x + 1 = 1000

2x = 999

x = 499.5

Check x = 499.5

log₁₀ (2 x 499.5 + 1)  = log₁₀ (1000) = 3 since 10³ = 1000

Sometimes when you solve logarithmic equations, you need to put all the logarithms on one side of the equation. Example #2 shows how to do this.

Example #2

log₅ (30x - 10) - 2 = log₅ (x + 6)

You can solve this one the same way you solved example #1.

Start by putting all logarithms on one side of the equation.

log₅ (30x - 10) - log₅ (x + 6) - 2 = log₅ (x + 6) - log₅ (x + 6)

log₅ (30x - 10) - log₅ (x + 6) -2 = 0

log₅ (30x - 10) - log₅ (x + 6) -2 + 2 = 0 + 2

log₅ (30x - 10) - log₅ (x + 6)  =  2

log₅ [ (30x - 10) / (x + 6) ] = 2

Now that it has the format that we want, we can just write it in exponential form.

30x - 10 / x + 6 = 5²

30x - 10 / x + 6 = 25       ( After multiplying both sides by x + 6 )

30x - 10 = 25 (x + 6)

30x - 10 = 25x + 150

30x - 25x = 150 + 10

5x = 160

x = 32

Check x = 32

log₅ (30x - 10) - 2 = log₅ (x + 6)

log₅ (30 x 32 - 10) - 2 = log₅ (32 + 6)

log₅ (960 - 10) - 2 = log₅ (32 + 6)

log₅ (950) - 2 = log₅ (38)

4.26 - 2 = 2.26

2.26 = 2.26

x = 32 is a good answer.

Now, you will learn how to solve logarithmic equations that have the following format

log_b X = log_b Y

To solve it, you just have to see that log_b X = log_b Y if and only if X = Y

More examples showing how to solve logarithmic equations using logarithmic properties.

Example #3

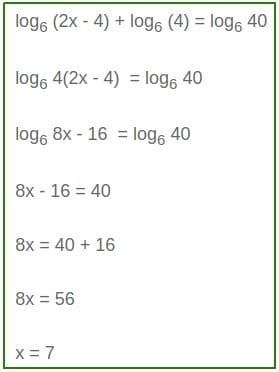
log₆ (2x - 4) + log₆ (4) = log₆ 40

log₆ 4(2x - 4)  = log₆ 40

log₆ 8x - 16  = log₆ 40

8x - 16 = 40

8x = 40 + 16

8x = 56

x = 7

Check x = 7

log₆ (2 x 7 - 4) + log₆ (4) = log₆ 40

log₆ (14 - 4) + log₆ (4) = log₆ 40

log₆ (10) + log₆ (4) = log₆ 40

log₆ (10 x 4) = log₆ 40

log₆ (40) = log₆ 40

x is 7 is a good answer.

Solve logarithmic equations with extraneous solutions 

Example #4

log₃ x + log₃ (x + 3)  = log₃ (2x + 6)

log₃ x(x + 3)  = log₃ (2x + 6)

log₃ (x² + 3x)  = log₃ (2x + 6)

x² + 3x  = 2x + 6

x² + 3x - 2x - 6 = 0

x² + x - 6 = 0

(x - 2) (x + 3) = 0

x = 2 and x  = -3

Check x = 2

log₃ 2 + log₃ (2 + 3)  = log₃ (2 x 2 + 6)

log₃ 2 + log₃ (5)  = log₃ (4 + 6)

 log₃ 2(5)  = log₃ (4 + 6)

log₃ (10)  = log₃ (10)

x  = 2  is a good answer.

Check x = -3

log₃ -3 + log₃ (-3 + 3)  = log₃ (2 x -3 + 6)

log₃ -3 + log₃ 0 = log₃ 0

Since  log₃ -3 does not exist, x  = -3 cannot be an answer. It is an extraneous solution.

Example #5

log (x + 2) + log (x - 1)  = 1

Use the product rule to rewrite the left side of the equation

log [(x + 2)×(x - 1)]  = 1

log [(x² - x + 2x - 2 )]  = 1

log [(x² + x - 2 )]  = 1

Notice that log₁₀ 10 = 1 since 10¹ = 10

log₁₀ [(x² + x - 2 )]  = log₁₀ 10

x² + x - 2 = 10

x² + x - 2 - 10 = 10 - 10

x² + x - 12 = 0

(x + 4)(x - 3) = 0

x = -4 and x = 3

Replace x = -4 and x = 3 into the original logarithmic equation to find the solution(s)

Check x = -4

log (x + 2) + log (x - 1)  = 1

log (-4 + 2) + log (-4 - 1)  = 1

log (-2) + log (-5)  = 1

log (-2) and log (-5) do not exist since the logarithm of a negative number does not exist.

Therefore, x = -4 is an extraneous solution.

Check x = 3

log (x + 2) + log (x - 1)  = 1

log (3 + 2) + log (3 - 1)  = 1

log (5) + log (2)  = 1

log(5×2) = 1

log 10 = 1

log₁₀ 10 = 1

Since log₁₀ 10 = 1 is a true statement, x = 3 is a solution.