Square roots table 

Below is a square roots table that can be used as a quick reference to check the square root of numbers from 1 through 100. It may be obvious yet see below how you can use the table to find the square root of a number.

N √N N √N
1

2

3

4

5

6

7

8

9

10

11

12

13

14

15

16

17

18

19

20

21

22

23

24

25

26

27

28

29

30

31

32

33

34

35

36

37

38

39

40

41

42

43

44

45

46

47

48

49

50

 1

1.414

1.732

2

2.236

2.449

2.646

2.828

3

3.162

3.317

3.464

3.606

3.742

3.873

4

4.123

4.243

4.359

4.472

4.583

4.690

4.796

4.899

5

5.099

5.196

5.292

5.385

5.477

5.568

5.657

5.745

5.831

5.916

6

6.083

6.164

6.245

6.325

6.403

6.481

6.557

6.633

6.708

6.782

6.856

6.928

7

7.071

 51

52

53

54

55

56

57

58

59

60

61

62

63

64

65

66

67

68

69

70

71

72

73

74

75

76

77

78

79

80

81

82

83

84

85

86

87

88

89

90

91

92

93

94

95

96

97

98

99

100

 7.141

7.211

7.280

7.348

7.416

7.483

7.550

7.616

7.681

7.746

7.810

7.874

7.937

8

8.062

8.124

8.185

8.246

8.307

8.367

8.426

8.485

8.544

8.602

8.660

8.718

8.775

8.832

8.888

8.944

9

9.055

9.110

9.165

9.220

9.274

9.327

9.381

9.434

9.487

9.539

9.592

9.644

9.695

9.747

9.798

9.849

9.899

9.950

10

How to read the square roots table to find the square root. 

Square root table

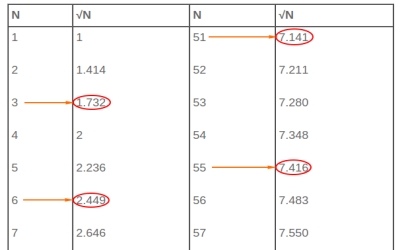
Above, we have copied a portion of the square roots table. From the table, you can clearly see the following square roots.

  • The square root of 3 is 1.732
  • The square root of 6 is 2.449
  • The square root of 51 is 7.141
  • The square root of 55 is 7.416
  • The square root of 7 is 2.646
  • The square root of 53 is 7.280
  • The square root of 4 is 2
  • The square root of 78 is 8.832
  • The square root of 31 is 5.568 

A brief review on what the square root of a number is.

The product of two identical numbers is another number. One of the two identical numbers is the square root of the other number.

For example, the product of 11 and 11 is another number or 121.

11 is the square root of 121.

Square root of a number