Standard deviation problems

Before we practice with some standard deviation problems, let us see how we can interpret the standard deviation.

Suppose a set of numbers has a mean (call it x) and all these numbers fall within 1 standard deviation of the mean.

Well, this is the range of values from one standard deviation below the mean to one standard deviation above the mean.

Mathematically, we can write: mean - s < x < mean + s

For example, if the mean is 40 and the standard deviation is 5, then a value x that is 1 standard deviation from the mean is in the range that you see below:

40 - 5 < x < 40 + 5

35 < x < 45

If the mean is 40 and the standard deviation is 5, then a value x that is 2 standard deviations from the mean is in the range you see below:

40 - 2 × 5 < x < 40 + 2 × 5

30 < x < 50

There is another way to interpret values for a standard deviation. It is called the empirical rule.

If a set of data has a distribution that is bell shape, then the following properties apply:

• About 68% of all the values fall within 1 standard deviation of the mean.

• About 95% of all the values fall within 2 standard deviations of the mean.

• About 99.7% of all the values fall within 3 standard deviations of the mean.

Now you are ready for a couple of standard deviation problems.

Problem #1:

Suppose the heights of men in a country have a bell-shaped distribution with a mean of 70 inches and a standard deviation of 3 inches.

1. What percentage of men have height between 61 inches and 79 inches?

2. What would this mean if someone's height is 3 standard deviation from the mean?

Solution:

1. The key to solving this problem is to see that 61 and 79 inches are each exactly 3 standard deviations from the mean.

3 × s = 3 × 3 = 9
70 - 9 = 61 and 70 + 9 = 79

Therefore, 99.7% of men have heights between 61 inches and 79 inches.

2. This could mean that the person is either very tall or very short.

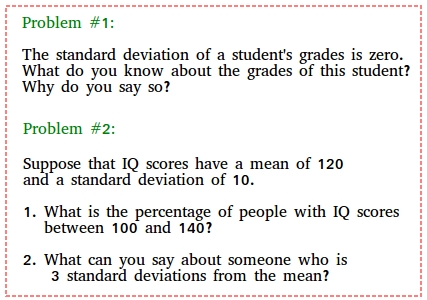
Problem #2:

Suppose that IQ scores have a mean of 120 and a standard deviation of 10.

1. What is the percentage of people with IQ scores between 100 and 140?

2. What can you say about someone who is 3 standard deviations from the mean?

Solution:

1.
100 is 2 standard deviations below the mean (100 = 120 - 2 × 10)

140 is 2 standard deviations above the mean (140 = 120 + 2 × 10)

95% of people have a score between 100 and 140

2. Somebody who is 3 standard deviations from the mean could either have a score of 90 or 160

The score of 90 will be considered low and somewhat average while the score of 160 is considered to be gifted.

Problem #3:

The standard deviation of a student's grades is zero. What do you know about the grades of this student? Why do you say so?

A. The student scored low grades.

B. The student scored the same grade every time.

C. The student's grade is unpredictable.

D. The student got a B on all tests.

Show answer