Volume of solids
How to calculate the volume of solids, such as the cube, the sphere, the cylinder, the pyramid, the cone, the ellipsoid, and the rectangular prism is what you will learn here.
As a warm up, take a look at the figure below looking like a pencil. How would you calculate the volume of the pencil above?
The volume of solids is a measure of the amount of space inside these figures.
Different figures lead to different computation.
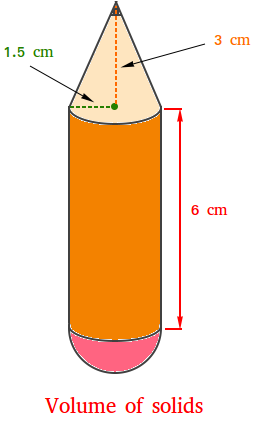
What important observation you need to make before calculating the volume of the pencil above? First, suppose that the pencil is made up of three different solids: a hemisphere, a cone, and a cylinder.
Therefore, volume of pencil = volume of cone + volume of cylinder + volume of hemisphere.
a. Volume of a cone = (π×r2×h) / 3
π = 3.14, r = 1.5 cm, and h = 3 cm
Volume of a cone = (3.14×1.52×3) / 3
Volume of a cone = (3.14×2.25×3) / 3
Volume of a cone = (21.195) / 3
Volume of a cone = 7.065 cm3
b. Volume of a cylinder = π×r2×h
π = 3.14, r = 1.5 cm, and h = 6 cm
Volume of a cylinder = 3.14×1.52×6
Volume of a cylinder = 3.14×2.25×6
Volume of a cylinder = 42.39 cm3
c. Volume of a sphere = (2×π×r3) / 3
π = 3.14 and r = 1.5 cm
Volume of a sphere = (2×3.14×1.53) / 3
Volume of a sphere = (6.28×1.53) / 3
Volume of a sphere = (6.28×3.375) / 3
Volume of a sphere = (21.195) / 3
Volume of a sphere = (21.195) / 3
Volume of a sphere = 7.065 cm3
volume of pencil = 7.065 cm3 + 42.39 cm3 + 7.065 cm3 = 56.52 cm3
More lessons showing how to calculate the volume of solids.
Other common figures where the volume is calculated are shown below
Volume of a cube
Learn how to compute the volume of a cube.
Volume of a box
Learn how to compute the volume of a box or rectangular prism.
Volume of a sphere
Learn how to get the volume of a sphere. We have also included a calculator that will help you to practice and to check your answers.
Volume of an ellipsoid
Learn how to compute the volume of an ellipsoid. This lesson shows also how to get a radius when the volume and two radii are given.
Volume of cylinders
Learn how to compute the volume of cylinders and use an interactive calculator to practice and check your answers.
Volume of a cone
Learn how to get the volume of a cone and practice with an interactive calculator.
Volume of a pyramid
Learn how to get the volume of a pyramid.
Volume of irregular shapes
Learn how to get the volume of irregular shapes.
Volume or capacity
A solid introduction of volume and capacity and how to choose the best unit to measure capacity.
|
Buy a comprehensive geometric formulas ebook. All geometric formulas are explained with well selected word problems so you can master geometry.  |
Recent Articles
-
How To Find The Factors Of 20: A Simple Way
Sep 17, 23 09:46 AM
There are many ways to find the factors of 20. A simple way is to... -
The SAT Math Test: How To Be Prepared To Face It And Survive
Jun 09, 23 12:04 PM
The SAT Math section is known for being difficult. But it doesn’t have to be. Learn how to be prepared and complete the section with confidence here.
100 Tough Algebra Word Problems.
If you can solve these problems with no help, you must be a genius!
