What is a Circle? Definition and Parts of a Circle
Interactive Lesson about the Circle and the Circle Parts
What is a Circle?
In geometry, a circle is the locus of points located at the same distance from a given fixed point.
The word locus sounds complicated, but it means set of all points on a plane. The fixed point is called center of the circle.
Another way then to define a circle is to say that it is the set of all points that are located at the same distance from a fixed point.
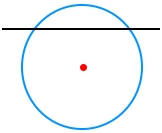
Looking at the figure above, all the blue dots are located at the same distance from the red dot (fixed point or center of the circle) and they form the locus of points mentioned above.
You can put a great number of blue dots until you get a closed curved line figure.The figure above shows a circle resulting from connecting the locus of all the points equidistant from the center.
What is a Circle in Real-Life? Real-Life Examples of Circle
- Bicycle wheels
- Dinner plates
- Coins such as dimes, quarters, nickels and pennies
- CDs and MP3 players
- Pizzas
- Darts
Below, we show a pizza and a dart as real-life examples of circles.
Dart and pizzaParts of a Circle and how to Name these Parts and the Lines that Cut a Circle?
Lines on a circle are given different names depending on how the line cuts the circle. There are five such lines.
- Chord
- Diameter
- Radius
- Secant
- Tangent
Chord of a Circle
A line segment joining two points on the circle is a chord. In other words, a chord is a segment whose endpoints are on the boundary of the circle. A chord usually divides a circle into two unequal halves unless the chord goes through the center of the circle. The following two red lines are examples of two chords.
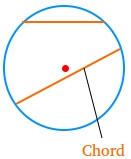Chord of a circleDiameter of a Circle
When a chord passes through the center of the circle, we call the chord a diameter. In other words, the longest chord of a circle is the diameter. A diameter usually divides a circle into two equal halves and each half is called a semi-circle. The following gray line is an example of a diameter.
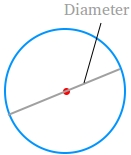Diameter of a circleRadius of a Circle
Half a diameter is called a radius. In other words, 2 radii = diameter as long as we are dealing with the same circle. The radius of a circle can also be defined as the distance between the center of the circle and any point on the circle. The following green line is the radius of the circle.
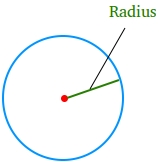Radius of a circleSecant of a Circle
A secant is a line that intersects a circle at two points. A secant of a circle is shown in black below.
Secant of a circleDid you see the difference between the secant and the other lines, especially the chord? The secant is not contained within the circle. It is extended beyond the loci (points on the boundary of the circle).
Tangent to a Circle
The tangent to a circle is a line that touches the boundary of the circle at exactly one point. If the line touches the boundary in two points, it is a chord as already stated, not a tangent!
The tangent of a circle is shown in red in the figure below. Notice how the red line touches the circle in exactly one point. The point where a circle and a tangent intersects is called the point of tangency and it is shown with a green dot.
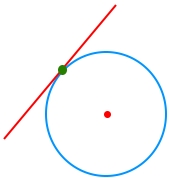Tangent to a circleArc of a Circle
An arc is a portion of the boundary of a circle. Suppose you put your finger on the boundary of the circle. If you move your finger on the boundary of the circle and the distance you cover is less than the perimeter of a the circle, what you end up with is an arc. In other words, if you use a compass and draw something less than 360 degrees or a full circle, then what you have drawn is an arc.
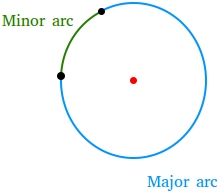
An arc can be a minor arc or a major arc as shown in the figure above. The minor arc is shown in green and the major arc is shown in blue.
Segments of a Circle
A segment of a circle is a portion of the circle created by a chord and either the minor arc or the major arc.
The figure above shows the segments of the circle. The one shown in purple is a minor segment and the one shown in turquoise is a major segment.
|
Buy a comprehensive geometric formulas ebook. All geometric formulas are explained with well selected word problems so you can master geometry.  |