Zero Product Property
Zero Product Property Quiz
Test your understanding of this fundamental algebraic concept
Quiz Summary
What is the Zero Product Property?
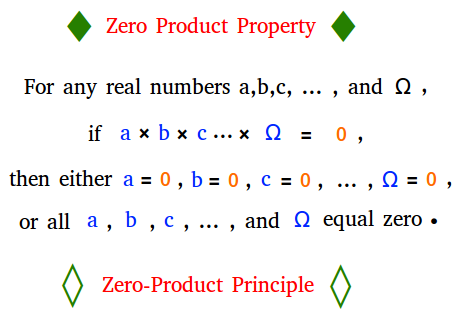
The zero product property, also called zero-product principle, states that for any real numbers a and b, if ab = 0, then either a equals zero, b equals zero, or both a and b equal zero. Since in many cases, there can be more than 2 factors, we can generalize the zero product property as shown below.
In other words, a product of factors is equal to zero if and only if any of the factors is equal to zero or up to all of the factors is equal to zero.
Example #1
If 6 × b = 0, then b must be zero since 6 is not equal to zero.
Example #2
If a × 10 = 0, then a must be zero since 10 is not equal to zero.
Example #3:
If a × b × 2 = 0, then we can have any of the following 3 situations:
0 × b × 2 = 0 ( a = 0 )
a × 0 × 2 = 0 ( b = 0 )
0 × 0 × 2 = 0 (a = 0 and b = 0)
The zero product property is frequently used to solve algebraic equations after writing them in factored form and then setting them equal to zero.
Using the Zero Product Property to Solve Quadratic equations
The zero product property is often used when solving quadratic equations by factoring.
Example #4
Solve x2 + 8x - 20 = 0
x2 + 8x - 20 = 0 (Original equation)
(x - 2)(x + 10) = 0 (Factored form)
x - 2 = 0 or x + 10 = 0 (Zero product property)
x = 2 or x = -10
The solutions of the equation are 2 and -10.
Using the Zero Product Property to Solve a more Complicated Equation
Example #5
Using the zero product property, solve the following equation for x. In order to help you focus on learning how to use the zero product property, the factored form of the equation is provided.
40x4 - 760x2 - 1200x = 0
The factored form of 40x4 - 760x2 - 1200x is equal to 10x(x + 2)(x - 5)(4x + 12)
10x(x + 2)(x - 5)(4x + 12) = 0
According the zero product property, 10x, (x + 2), (x - 5), and 4x + 12 are all equal to zero.
a. 10x = 0
Since 10 times 0 = 0, x = 0.
b. x + 2 = 0
x + 2 - 2 = 0 - 2
x + 0 = -2
x = -2
c. x - 5 = 0
x - 5 + 5 = 0 + 5
x + 0 = 0 + 5
x = 5
d. 4x + 12 = 0
4x + 12 - 12 = 0 - 12
4x + 0 = -12
4x = -12
4x/4 = -12/4
x = -3
The solutions to the equation 40x4 - 760x2 - 1200x = 0 are -3, -2, 0, and 5.
Does the Zero Product Property Work for Matrices as Well?
The zero product property does not work for the multiplication of matrices since the product of two non-zero matrices can be equal to zero.
$$ A = \begin{bmatrix} 0 & 0\\ 3 & 6\\ \end{bmatrix} B = \begin{bmatrix} 0 & -2\\ 0 & 1\\ \end{bmatrix} $$$$ A × B = \begin{bmatrix} 0 × 0 + 0 × 0 & 0 × -2 + 0 × 1\\ 3 × 0 + 6 × 0 & 3 × -2 + 6 × 1\\ \end{bmatrix} $$
$$ A × B = \begin{bmatrix} 0 + 0 & 0 + 0\\ 0 + 0 & -6 + 6\\ \end{bmatrix} $$
$$ A × B = \begin{bmatrix} 0 & 0\\ 0 & 0\\ \end{bmatrix} $$
Notice that when you multiply the two matrices A and B, you end up with a zero matrix. However, neither A nor B is a zero matrix. A and B do not have to be zero matrices.